Video After Video in the Post-Media Age (Monday, May 12 - Friday, May 16, 2008).
Science as Navigation: Leonhard Euler's Journeys. Symposium on the Occasion of Euler's 300th birthday (November 30, 2007)
Calculating Images. Representation by Algorithm in Science and Art (March 4-5, 2005)
Packrats and Bureaucrats: Study in the Archive (February 5-6, 2001)
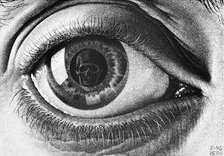
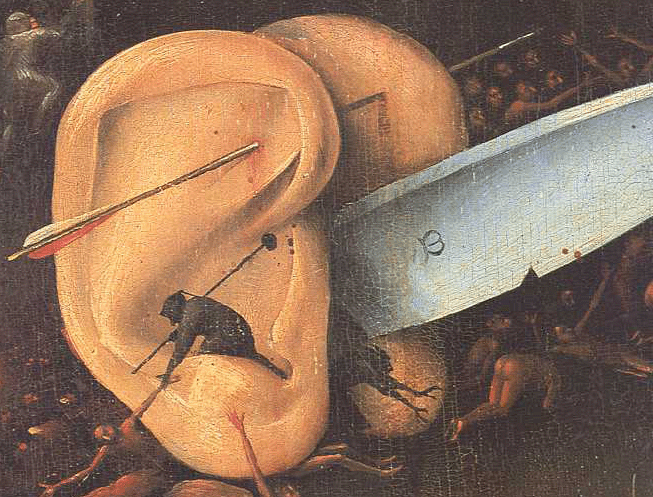
Eye or Ear: Walter Benjamin on Optical and Acoustical Media
Conference at the University of California, Santa Barbara
Free and open to the public
December 1, 2006
10:30 am to 6:00 pm
Humanities & Social Sciences Building, 6th floor,
McCune Conference Room
_________________________________________
Description | Program | Directions | Contact
Description
Our conference investigates the relationship between three aspects of Benjamin’s work: his language theory, his media theory, and his radio praxis. Since the essay “The Work of Art in the Age of Mechanical Reproduction” is predominantly focused on painting, photography and film, much more is known about Benjamin’s understanding of these optical media than about his approach to such acoustic devices as the telephone, the wireless telegraph, radio, and sound film. Consequently, it is often forgotten that Benjamin reads both words and images as repetitions of the divine act of breathing, hence, as a reenactment of the biblical creation myth, and that, at the time when the artwork essay was published, sound film was well under way.
Program
| 10:30 am | Welcome |
| Henry T. Yang, Chancellor | |
|
Elisabeth Weber, Chair, Department of Germanic, Slavic and Semitic Studies |
|
| 10:45 am | Wolfgang Hagen, Humboldt Universität, Berlin |
| “On the Minute” – Benjamin’s Silent Work for the German Radio | |
| 11:30 am | Rob Ryder, Northwestern University |
| Shell-Shock: Sounding the Acoustic Unconscious | |
| 12:30 pm | Lunch and coffee break |
| 2:00 pm | Wolf Kittler, University of California, Santa Barbara |
| Benjamin’s Aura: Breathing, Seeing, Listening | |
| 2:45 pm | Michael Levine, Rutgers University |
| Eavesdropping on Tradition: Benjamin’s Reading of Kafka | |
| 3:30 pm | Bettine Menke, Universität Erfurt and University of California, Santa Barbara, Max Kade Visiting Professor |
| Echo(es): The Evanescent Presence of Sound in Benjamin’s Texts | |
| 4:30 pm | General discussion |
| 5:15-6:15 | Reception |
Directions
The Humanities and Social Sciences Building (HSSB) is located next to the Events Center, off Ocean Road. Park in lot 27 or 21 in a “C” space. Parking permits must be displayed at all times and may be purchased from permit dispensers located in every parking lot; permits are payable by Visa, MasterCard, student Access card or cash.
For More Information
Contact Sven Spieker at spieker@gss.ucsb.edu or Elisabeth Weber at weber@gss.ucsb.edu
SCIENCE AS NAVIGATION: LEONHARD EULER'S JOURNEYSAn International Conference at UC Santa Barbara on the Occasion of Leonhard Euler’s 300th Birthday November 30, 2007; 9:00 am - 7:00 pm
Detailed Presentation Topics
Brian Hopkins (St. Peter’s College, NJ), “The Beginning of Graph Theory” Wladimir Velminski ( Humboldt University, Berlin), “ Leonhard Euler’s Strategies of Visualization” Matt Wickman ( Brigham Young University, UT), “ Burns's Nature, Euler's Path” Julian Havil (Winchester/UK), “Euler: the Basel Problem, the Polyhedral Formula and 'Proof' ” Graph theory is a comparably young area of mathematics where relations of adjacency are arbitrary and standard measures from geometry are irrelevant. Euler discussed two problems now considered to be within the purview of graph theory: the bridges of Königsberg and knight's tours. The primary topic in this talk will be the puzzle about seven bridges that spanned the Pregel River. In addition to exploring Euler's solution to the problem (different from today's treatment), we will consider his correspondence preceding the article: Euler called the problem "banal," so why did he bother solving it? Euler's contribution to the recreational mathematics study of chessboard problems seemed unrelated to the bridges in both setting and solution method, but we will sketch the development of graph theory to sufficient generality that encompasses both topics. I will explore the relationship between Euler’s thought and the Scottish Enlightenment, particularly as it involves the theory of attraction. Specifically, I will discuss an often-overlooked aspect of Euler’s work - his metaphysics (e.g. of ether as a quasi-material, half-spiritual fluid filling the universe), relative to the Scottish Enlightenment theory of sympathy. Some might argue that scholars ever since Daniel Bernoulli have disregarded Euler’s metaphysics with good reason. My aim here is less to rehabilitate Euler’s “ethereal” thought than to indicate its provocative correspondence with Scottish moral philosophy and popular poetry. Ultimately, I will suggest that “attraction” in these contexts distills an atmospheric quality which resembles and partly foreshadows a modern concept of environment. I propose to point out the difficulties inherent in the concept of rigorous mathematical proof and indicate what 'proof' meant in the 18th century and most particularly, to Euler. I will show a couple of simple examples of divergent series with which he struggled and move to his famous Basel Problem result, again indicating the difference between the modern view of acceptability of proof and that of Euler et al. I will then move to Polyhedral Formula and reinforce the point; also, I should like to use the formula to establish two great results (each in a couple of lines) as an indication of its power: the first, that there at most 5 Platonic solids and the second a result that is central to any proof of The names of those Euler wrote to and of those who wrote to him are most of the significant mathematicians and physicists, philosophers and metaphysicians, theologians and apologists, aristocrats and monarchs. His letters place Euler at the scientific epicenter for the half-century spanning 1727-1783. Euler was aware that, irrespective of where the correspondence was going and to whom it was addressed, the content of the letters constituted a focused direction. These letters were not papers but rather documents that provided the initial scope of a range of subjects and interests, and they are to be seen as the continuous integration of scientific, philosophical, pedagogical and religious knowledge in discrete contributions that reveal the continuum of knowledge. I will review the history of the theories concerning the shape of the Earth, beginning with a glance at Aristotle (De Caelo, II.13, 14), who gave various arguments to support the view that the Earth was In 1727 the young Euler arrived in St. Petersburg to take up his position as adjunct in the Petersburg Academy. (He was promoted to fill Bulfinger's position as Professor of Physics in 1731 and took Daniel Bernoulli's position as Professor of Mathematics in 1733.) Between 1728 and 1742 the Academy published a "supplement" to the St. Petersburg Gazette, called "Notes to the Gazette", which contained scientific articles written at a popular level by members of the Academy. It was published simultaneously in Russian and in German. Until 1738 the articles appeared anonymously; thereafter the author was indicated by an initial. The Russian scholar Judith Kopelevich has suggested that some of the early, anonymous articles were probably by Euler, in whole or in part. In 1738, the year following Maupertuis' return from the Lapland expedition, Euler published in this journal an article "On the Shape of the Earth". This was the Euler's only contribution to the "Notes" which was known to Gustav Enestrom, who listed it as item 32 in his catalogue of Euler's works. (Enestrom in fact knew only the Russian version of the article.) J. J. Burckhardt, who edited the German version of this article for Euler's Opera Omnia, describes it as "a small masterpiece of Euler's expository art, [one that] will afford pleasure to every reader". In this article, Euler explains the scientific issues involved, and reviews the various means by which the true shape of the Earth can be determined. Curiously, Euler adduces as one reason for trusting the results of the Lapland expedition the circumstance that the members of the expedition had previously been strong opponents of the Newtonian view concerning the shape of the Earth. Actually, Maupertuis had become a convinced Newtonian after a visit to London in 1728, and in 1735 had published a memoir "Sur la figure de la terre" supporting the Newtonian view. (In a historical irony, Maupertuis would a few years later become Euler's boss at the Berlin Academy.) In my talk, I will not try to describe everything Euler does in this article. One thing that I want to look at particularly, however, is Euler's analysis of the experimental errors in the measurements made by the expedition, in order to decide whether the results were sufficiently accurate to settle the question of the shape of the Earth. Euler certainly had a good opinion of the capabilities of his St. Petersburg readers! Based on Euler's journals and his autobiography, I examine Euler's constructions of visualization with a special focus on topological ideas. From the very beginning of his life, Euler was chained to mathematics. Thus, the written contemplation of the past approaches calculatory graphs. While Euler devoted himself to calculus professionally, he occasionally made discoveries. In one case this took the form of "Rösselsprungproblem" - the problem of the chess board with only one knight left.
Contact Sven Spieker at spieker@gss.ucsb.edu. 6206 Phelps Hall University of California Santa Barbara, CA 93106-4130 805-893-2131 / Fax: 805-893-2374 www.gss.ucsb.edu
University of California, Santa Barbara
|


